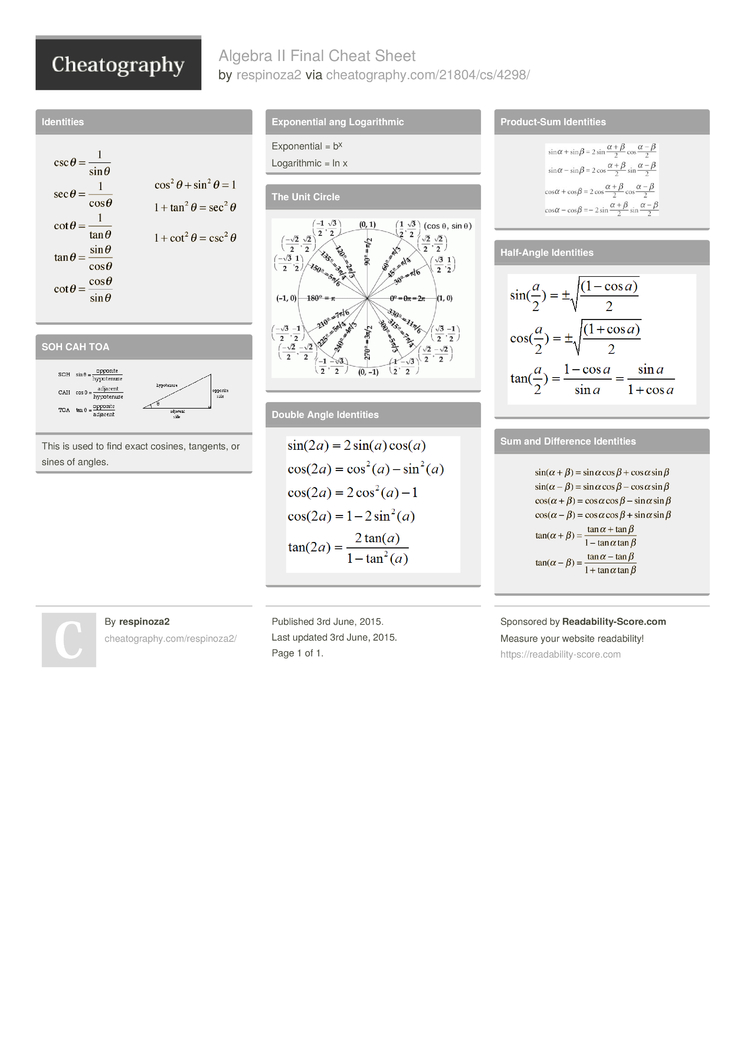
Is Algebra the cornerstone of modern mathematics? Absolutely, it is. Algebra forms the backbone of mathematical understanding and serves as a gateway to advanced concepts in various fields such as physics, engineering, computer science, and economics. The ability to manipulate symbols, solve equations, and understand functions is not just an academic exercise but a practical skill that empowers problem-solving across disciplines. As students delve into algebraic studies, they encounter tools like cheat sheets, reference guides, and conversion charts that simplify complex topics. These resources are invaluable for mastering fundamental properties, formulas, and techniques essential for success in exams and real-world applications.
Algebra's significance extends beyond high school classrooms. It plays a crucial role in standardized tests like the Algebra 2 Regents Exam, where familiarity with key concepts can make or break performance. For instance, quadratic equations, polynomial identities, and exponential functions often appear on these assessments. Moreover, college-level courses frequently build upon foundational algebraic principles, making proficiency in this area indispensable for higher education. Even professionals rely on algebra daily—engineers use it to design structures, economists apply it to model markets, and data scientists leverage it to analyze trends. Thus, whether preparing for finals or advancing careers, having access to concise yet comprehensive study aids becomes paramount.
| Name | Dr. Rossi |
|---|---|
| Place of Birth | Italy |
| Date of Birth | January 15, 1970 |
| Education | Ph.D. in Mathematics from Stanford University |
| Current Position | Professor of Mathematics at MIT |
| Specialization | Linear Algebra & Abstract Algebra |
| Awards | Recipient of the Fields Medal (2020) |
| Notable Publications | List of Publications |
The realm of algebra encompasses diverse subfields, each offering unique challenges and opportunities. Linear algebra focuses on vector spaces and linear transformations, providing tools vital for machine learning algorithms and graphics rendering. Meanwhile, abstract algebra explores algebraic structures like groups, rings, and fields, underpinning cryptography and coding theory. Students navigating these areas benefit immensely from structured resources tailored to specific needs. Cheat sheets summarizing critical formulas, step-by-step problem-solving strategies, and common pitfalls serve as lifelines during intense study sessions. Furthermore, interactive platforms enabling practice through quizzes and simulations enhance engagement and retention.
Consider the example of factoring cubic polynomials—a topic frequently encountered in both Algebra I and II curricula. Expressions like \(x^3 + a^3\) and \(x^3 - a^3\) follow distinct patterns based on sum/difference of cubes formulae: \(x^3 + a^3 = (x + a)(x^2 - ax + a^2)\) and \(x^3 - a^3 = (x - a)(x^2 + ax + a^2)\). Similarly, recognizing factorizations involving even powers, such as \(x^{2n} - a^{2n} = (x^n - a^n)(x^n + a^n)\), proves beneficial when tackling higher-degree equations. Mastery over such techniques not only simplifies computations but also fosters deeper conceptual clarity. Additionally, being aware of special cases, e.g., odd exponents allowing further decomposition (\(x^n - a^n = (x - a)(x^{n-1} + ax^{n-2} + ... + a^{n-1})\)), adds versatility to one's toolkit.
In preparation for comprehensive evaluations like final exams, students often turn to review materials encompassing broad spectrums of algebraic knowledge. An effective approach involves combining multiple sources—textbooks, online tutorials, instructor notes—to create personalized summaries addressing individual strengths and weaknesses. For instance, incorporating visual representations alongside textual explanations caters to varied learning styles while reinforcing memory recall. Likewise, practicing past papers under timed conditions simulates actual testing environments, thereby reducing anxiety and boosting confidence levels.
CLEP examinations represent another avenue where algebra expertise translates directly into academic credit. Specifically designed to assess mastery equivalent to introductory college courses, these standardized tests reward candidates who demonstrate thorough comprehension coupled with efficient application skills. College Algebra CLEP specifically targets single-variable topics including linear/quadratic equations, inequalities, functions, matrices, and sequences/series. Leveraging official study guides supplemented by community-generated tips enhances chances of achieving desired scores significantly.
For those seeking supplementary support outside traditional classroom settings, digital repositories brimming with curated content prove invaluable. Websites dedicated exclusively to algebra instruction offer everything from basic property refreshers to intricate proof walkthroughs. One notable collection comprises thirty-six additional references ranging from concise cheat sheets targeting specific units to exhaustive manuals delving deeply into theoretical aspects. Such diversity ensures learners find precisely what suits their requirements without unnecessary clutter. Noteworthy mentions include Algebra 1 Final Exam Review with Answers, Linear Algebra Practice Final Exam with Solutions, and Algebra II Conversion Chart—each catering uniquely to different stages along the educational journey.
Finally, community involvement amplifies learning outcomes exponentially. Platforms facilitating peer interaction allow sharing insights, discussing ambiguities, and celebrating achievements collectively. Reddit threads featuring user-submitted contributions exemplify collaborative spirit thriving within niche interest groups. Take, for example, the post titled Not the Prettiest But My Linear Algebra Cheat Sheet for My Exam :) wherein redditor pxuline shared homemade notes garnering overwhelming positive feedback. Instances like these underscore importance of building supportive networks fostering continuous growth and improvement.
| Topic | Formula | Description |
|---|---|---|
| Sum/Difference of Cubes | \(x^3 \pm a^3 = (x \pm a)(x^2 \mp ax + a^2)\) | Factorization method applicable to third-degree binomials. |
| Difference of Squares | \(x^2 - a^2 = (x - a)(x + a)\) | Simplifies expressions containing two perfect squares separated by subtraction. |
| Exponential Growth/Decay | \(A = P(1 + r)^t\) or \(A = Pe^{rt}\) | Models population dynamics, investment returns, radioactive decay processes. |
| Quadratic Formula | \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\) | Solves second-degree polynomial equations regardless of discriminant value. |
| Logarithmic Identity | \(\log_b(xy) = \log_b(x) + \log_b(y)\) | Enables breaking down products inside logarithms into sums. |